Information Theory SG by Dr. Ryusuke Hamazaki on December 16, 2020
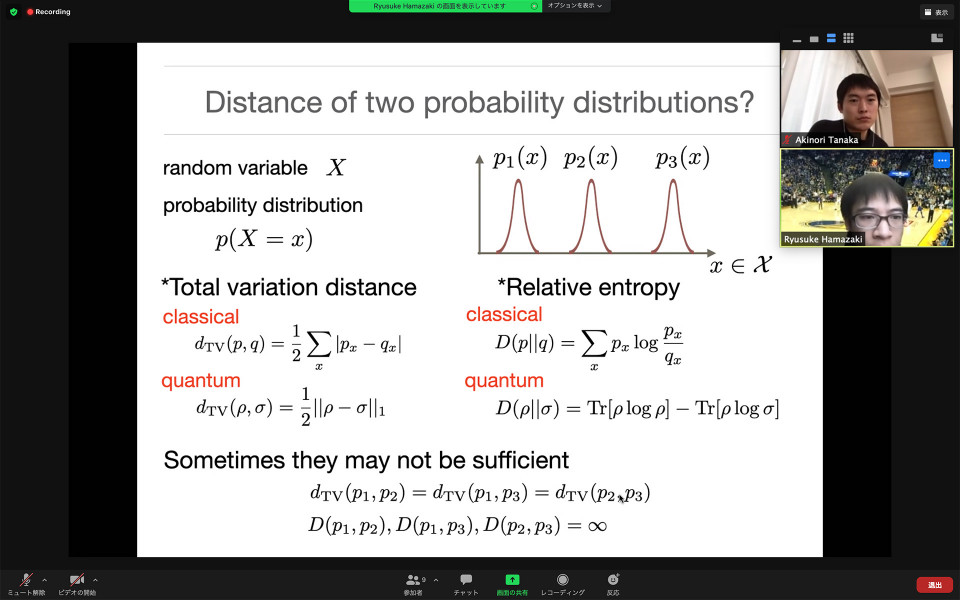
In the journal club of Information Theory Study Group held on December 16th, Dr. Ryusuke Hamazaki (CPR/iTHEMS) gave us a talk about recent proposals of a quantum version of the Wasserstein distance. He first reviewed the commonly used quantities expressing the distance between probability distributions, i.e., the total variation distance and the relative entropy. After pointing out certain problems with these quantities, he introduced the classical Wasserstein distance of order 1 as a better definition of the distance and explained its representative properties like transportation inequality and tensorization property. Next, he introduced a quantum counterpart of the Wasserstein distance, which can apply to spin-1/2 quantum systems. In contrast to the total variation distance, the quantum Wasserstein distance has interesting properties like the invariance under permutation or local unitary transformation. Lastly, he explained an application of the quantum version of transportation inequality to the eigenvalue distribution. Reflecting the interdisciplinary subject of the talk, there were several questions and comments from both mathematical and physical viewpoints. We are grateful to Ryusuke for the great talk!
Kyosuke Adachi (BDR/iTHEMS)