Math Seminar by Dr. Takahiro Kitayama on November 16, 2020
On November 16, professor Takahiro Kitayama from University of Tokyo gave a talk entitled “Representations of fundamental groups and 3-manifold topology” at the iTHEMS math seminar.
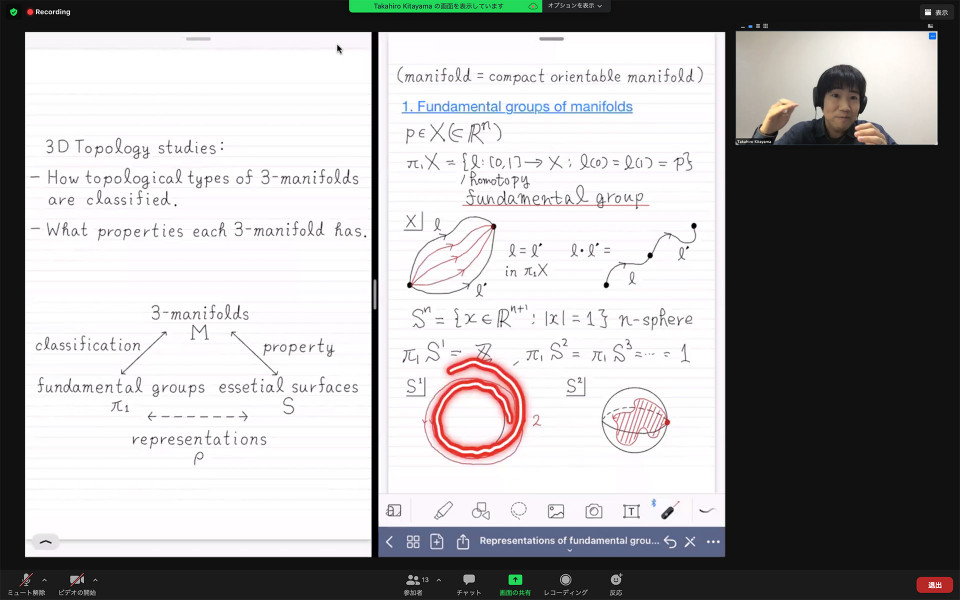
In the first part, he introduced a central motivation of 3-manifold topology: classify all 3-manifolds up to diffeomorphisms. As one of the important tools, he introduced the fundamental groups of spaces. He reviewed several known results of the fundamental groups of manifolds. Next, he focused on essential surfaces and introduced Haken 3-manifolds as an important class of 3-manifolds. In particular, he introduced several examples of Haken and non-Haken manifolds. At the end of the first talk, he explained SL(2,C)-representation spaces (character varieties) of the fundamental groups of 3-manifolds. He mentioned that the representation space has been used as a fundamental tool to classify knots and 3-manifolds.
In the second part, he first mentioned Culler-Shalen, Morgan-Shalen’s theorem which says that an ideal point of the SL(2,C)-character variety of a given 3-manifold M gives an essential surface of M. Friedl, Hara, Kitayama, and Nagel developed C-S and M-S’s theory for the Lie group SL(n,C). He explained the main idea to obtain all essential surfaces from ideal points of SL(n,C)-character variety. In particular, he introduced the tautological representation depending on some affine curve of the SL(2,C)-character variety, and an action of the fundamental group on some contractible simplicial complex called the Bruhat-Tits building. Then by a standard argument of topology, he constructed some simplicial map f from the universal covering space of a given 3-manifold to the Bruhat-Tits building. By taking the inverse image of f(after ignoring trivial parts and dividing by the fundamental group of the 3-manifold), he finally constructed an essential surface. Next, he also told us about a relation between (homotopy types of) boundary loops of essential surfaces of knot complements and slopes of sides of the Newton polygon obtained from A-polynomials. He said an essential idea of the result, which can detect whether the boundary of an essential surface obtained from an ideal point is boundary parallel or not. At the end of the second talk, as the leading coefficients of torsion functions, he gave a function c_{M, ψ} on the SL(n, C)-character variety. After explaining Dunfield-Friedl-Jackson’s conjecture, he gave a partial solution of the conjecture which is related to the finiteness of c_{M, ψ} on the ideal points.